how to find the domain of a logarithmic function

Logarithmic Functions

The logarithmic function is an important medium of math calculations. Logarithms were discovered in the xvi^th century by John Napier a Scottish mathematician, scientist, and astronomer. Information technology has numerous applications in astronomical and scientific calculations involving huge numbers. Logarithmic functions are closely related to exponential functions and are considered as an changed of the exponential function. The exponential function a^x = N is transformed to a logarithmic function log_aDue north = 10.

The logarithm of any number N if interpreted as an exponential course, is the exponent to which the base of the logarithm should be raised, to obtain the number N. Here we shall aim at knowing more than about logarithmic functions, types of logarithms, the graph of the logarithmic function, and the backdrop of logarithms.

1.	What are Logarithmic Functions?
2.	Domain and Range of Log Functions
iii.	Logarithmic Graph
four.	Graphing Logarithmic Functions
5.	Properties of Logarithmic Functions
6.	Derivative and Integral of Logarithmic Functions
7.	FAQs on Logarithmic Functions

What are Logarithmic Functions?

The bones logarithmic function is of the form f(x) = log_a10 (r) y = log_ax, where a > 0. It is the inverse of the exponential function a^y = x. Log functions include natural logarithm (ln) or common logarithm (log). Here are some examples of logarithmic functions:

• f(ten) = ln (x - 2)
• g(x) = log₂ (x + v) - ii
• h(x) = two log x, etc.

Some of the non-integral exponent values tin be calculated easily with the use of logarithmic functions. Finding the value of x in the exponential expressions 2^x = 8, 2^x = xvi is easy, simply finding the value of 10 in 2¹⁰ = ten is hard. Here we tin can use log functions to transform ii^x = 10 into logarithmic grade every bit log₂10 = x and and so detect the value of x. The logarithm counts the number of occurrences of the base in repeated multiples. The formula for transforming an exponential function into a logarithmic function is equally follows.

The exponential function of the grade a^x = N can be transformed into a logarithmic office log_aNorth = x. The logarithms are generally calculated with a base of 10, and the logarithmic value of whatsoever number can exist constitute using a Napier logarithm tabular array. The logarithms can be calculated for positive whole numbers, fractions, decimals, but cannot exist calculated for negative values.

Domain and Range of Log Functions

Permit us consider the basic (parent) common logarithmic function f(ten) = log x (or y = log x). We know that log x is defined but when ten > 0 (effort finding log 0, log (-ane), log (-2), etc using your computer. You will come up with an error). So the domain is the set of all positive existent numbers. At present, we will find some of the y-values (outputs) of the function for different x-values (inputs).

• When x = 1, y = log 1 = 0
• When x = 2, y = log 2 = 0.3010
• When x = 0.2, y = -0.6990
• When 10 = 0.01, y = -ii, etc

We can see that y can be either a positive or negative real number (or) it tin can be zero as well. Thus, y can take the value of any existent number. Hence, the range of a logarithmic office is the set up of all existent numbers. Thus:

• The domain of log function y = log ten is x > 0 (or) (0, ∞).
• The range of any log function is the set of all real numbers (R)

Case: Find the domain and range of the logarithmic function f(x) = 2 log (2x - iv) + 5.

Solution:

For finding domain, set the argument of the role greater than 0 and solve for x.

2x - iv > 0
2x > 4
x > ii

Thus, domain = (2, ∞).

As we have seen earlier, the range of any log role is R. So the range of f(x) is R.

Logarithmic Graph

We have already seen that the domain of the basic logarithmic function y = log_a 10 is the set of positive existent numbers and the range is the set of all real numbers. Nosotros know that the exponential and log functions are inverses of each other and hence their graphs are symmetric with respect to the line y = ten. Too, notation that y = 0 when ten = 0 as y = log_a1 = 0 for any 'a'. Thus, all such functions have an x-intercept of (1, 0). A logarithmic function doesn't have a y-intercept as log_a0 is not defined. Summarizing all these, the graphs of exponential functions and logarithmic graph look like below.

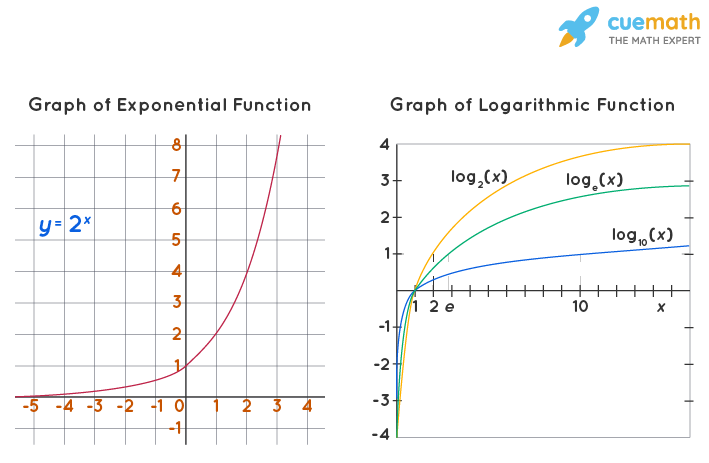
Backdrop of Logarithmic Graph

• a > 0 and a ≠ one
• The logarithmic graph increases when a > one, and decreases when 0 < a < 1.
• The domain is obtained by setting the argument of the function greater than 0.
• The range is the ready of all real numbers.

Graphing Logarithmic Functions

Earlier cartoon a log function graph, only have an idea of whether you get an increasing bend or decreasing curve as the respond. If the base of operations > 1, then the curve is increasing; and if 0 < base < 1, then the curve is decreasing. Here are the steps for graphing logarithmic functions:

• Find the domain and range.
• Notice the vertical asymptote by setting the statement equal to 0. Note that a log office doesn't accept any horizontal asymptote.
• Substitute some value of x that makes the argument equal to i and use the property log_a 1 = 0. This gives the states the x-intercept.
• Substitute some value of x that makes the argument equal to the base and utilise the holding log_a a = one. This would give usa a signal on the graph.
• Bring together the two points (from the last ii steps) and extend the bend on both sides with respect to the vertical asymptote.

Example: Graph the logarithmic part f(x) = 2 log_three (x + one).

Solution:

Here, the base of operations is 3 > 1. So the curve would be increasing.

For domain: x + one > 0 ⇒ ten > -1. So domain = (-one, ∞).

Range = R.

Vertical asymptote is 10 = -1.

• At x = 0, y = 2 log₃ (0 + i) = two log₃ 1 = two (0) = 0
• At ten = two, y = 2 log_three (two + 1)= two log₃ 3 = 2 (ane) = 2

If we want more clarity, we tin can form a table of values with some random values of x and substitute each of them in the given function to compute the y-values. This way, we get more points on the graph and it helps in getting the perfect shape of the graph.

Thus, (0, 0) and (2, 2) are two points on the curve. Thus, the log role graph looks every bit follows.

Properties of Logarithmic Functions

Logarithmic role properties are helpful to work across complex log functions. All the general arithmetic operations across numbers are transformed into a different prepare of operations within logarithms. The product of two numbers, when taken inside the logarithmic functions is equal to the sum of the logarithmic values of the two functions. Similarly, the operations of division are transformed into the difference of the logarithms of the two numbers. Let us list the of import backdrop of log functions in the below points.

• log ab = log a + log b
• loga/b = log a - log b
• log_ba = (log_c a)/(log_c b) (change of base rule)
• loga^x = 10 loga
• log_a i = 0
• log_a a = 1

Derivative and Integral of Logarithmic Functions

The derivation of the logarithmic office gives the slope of the tangent to the curve representing the logarithmic office. The formula for the derivative of the mutual and natural logarithmic functions are as follows.

• The derivative of ln ten is 1/x. i.e., d/dx. ln x = i/x.
• The derivative of logₐ x is i/(x ln a). i.e., d/dx (logₐ x) = 1/(10 ln a).

The integral formulas of logarithmic functions are as follows:

• The integral of ln ten is ∫ ln x dx = ten (ln x - i) + C.
• The integral of log x is ∫ log ten dx = x (log 10 - one) + C.

Related Topics:

• Exponents
• Exponent Rules
• Properties of Logarithms
• Logs in calculations

Solved Examples on Logarithmic Functions

1. Example 1: Limited 4³ = 64 in logarithmic grade.

   Solution: The exponential grade a^x = N tin be written in logarithmic office form as log_aN = x .

   Hence, 4³ = 64 can be written in logarithmic form as log₄64 = 3.

   Answer: log₄64 = three

2. Example 2: Simplify log_two (1/128).

   Solution: We use the properties of logarithmic office to simplify the given logarithm.

   log₂ (1/128) = log_ii ane - log_two 128

   = 0 - log_two 2⁷

   = -log₂ ii⁷

   = -vii log_two ii

   = -7 (1)

   = -vii

   Answer: Hence log₂ (1/128) = -7

3. Example 3: Find the domain, range, vertical and horizontal asymptotes of the logarithmic role f(x) = 3 log₂ (2x - three) - vii.

   Solution:

   For domain, 2x - 3 > 0 ⇒ x > three/2. Hence domain = (iii/2, ∞).

   The range of any log function is (-∞, ∞).

   For vertical asymptote (VA), 2x - 3 = 0 ⇒ x = 3/2.

   A logarithmic graph never has a horizontal asymptote (HA).

   Answer: Domain = (3/two, ∞); Range = (-∞, ∞); VA is x = 3/ii; No HA.

get to slidego to slidego to slide

Breakdown tough concepts through unproblematic visuals.

Math volition no longer exist a tough subject, peculiarly when you understand the concepts through visualizations.

Volume a Free Trial Class

Do Questions on Logarithmic Functions

go to slidego to slide

FAQs on Logarithmic Functions

How to Solve Logarithmic Functions?

The logarithmic role tin be solved using the logarithmic formulas. The product of functions inside logarithms is equal (log ab = log a + log b) to the sum of two logarithm functions. The sectionalisation of two logarithm functions(loga/b = log a - log b) is changed to the divergence of logarithm functions. The logarithm functions can too be solved by changing it to exponential class.

How to Graph Logarithmic Functions?

The graph of log function y = log x tin exist obtained by finding its domain, range, asymptotes, and some points on the curve. To find some points on the curve nosotros can use the following backdrop:

• log ane = 0
• log 10 = 1

What are Asymptotes of a Logarithmic Function?

Here are the asymptotes of a logarithmic function f(x) = a log (x - b) + c:

• The vertical asymptote is ten = b.
• There is no horizontal asymptote.

How Are Exponential and Logarithmic Functions Related?

The exponential office of the class a^x = N can be transformed into a logarithmic role log_aN = ten. Here the exponential functions 2^x = 10 is transformed into logarithmic form equally log_ii10 = ten, to find the value of 10. The logarithm counts the numbers of occurrences of the base of operations in repeated multiples.

What is the Difference Between Natural Logarithmic and Common Logarithmic Functions?

The logarithmic functions are broadly classified into two types, based on the base of operations of the logarithms. We have natural logarithms and common logarithms. Natural logarithms are logarithms to the base 'e', and mutual logarithms are logarithms to the base of operations of 10. Further logarithms can be calculated with reference to whatever base, just are frequently calculated for the base of either 'e' or '10'. The natural logarithms are written as log_ex (or) ln x, and the common logarithms are written as log_tenx (or) log 10. To obtain the value of 10 from natural logarithms, it is equal to the power to which due east has to be raised to obtain x.

• e = ii.718
• log_eN = 2.303 ×log_xNorth
• log₁₀N = 0.4343 × log_eastNorth

The value of e = 2.718281828459, but is often written in short equally e = 2.718. Too, the higher up formulas help in the interconversion of natural logarithms and mutual logarithms.

How to Differentiate Logarithmic Functions?

The differentiation of a logarithmic function results in the changed of the function. The differentiation of ln 10 is equal to i/x. (d/dx .ln x = ane//x). Besides, the antiderivative of ane/x gives back the ln function.

What Is the Range of Logarithmic Functions?

The range of a logarithmic part takes all values, which include the positive and negative real number values. Thus the range of the logarithmic function is from negative infinity to positive infinity.

What Is the Domain of Logarithmic Functions?

The logarithms can be calculated for positive whole numbers, fractions, decimals, but cannot be calculated for negative values. Hence the domain of the logarithmic function is the set up of all positive real numbers.

What is the Formula for Logarithmic Functions?

The following formulas are helpful to work and solve the log functions.

• log ab = log a + log b
• loga/b = log a - log b
• log_ba = (log a)/(log b)
• loga¹⁰ = ten loga

What Are Logarithmic Functions Used For?

Logarithmic functions have numerous applications in physics, engineering science, astronomy. The numeric measurements in astronomy include huge numbers with decimals and exponents. The huge scientific calculations tin be easily simplified and calculated using log functions. The logarithmic functions help in transforming the product and division of numbers into sum and difference of numbers.

Source: https://www.cuemath.com/algebra/logarithmic-functions/

Posted by: polkconat1975.blogspot.com

Share this post